What App Does The Odd Ones Out Use To Draw

Even and Odd Functions

You may exist asked to "determine algebraically" whether a function is even or odd. To do this, you take the function and plug –x in for x , and then simplify. If you end up with the exact same office that you started with (that is, if f(–x) = f(x), and so all of the signs are the same), then the function is fifty-fifty. If you end upward with the exact opposite of what yous started with (that is, if f(–x) = –f(x), so all of the signs are switched), then the role is odd.

In all other cases, the function is "neither fifty-fifty nor odd".

Let'due south see what this looks like in activeness:

Content Continues Below

• Make up one's mind algebraically whether f(10) = –310 ² + four is fifty-fifty, odd, or neither.

If I graph this, I will come across that this is "symmetric most the y -axis"; in other words, whatever the graph is doing on 1 side of the y -centrality is mirrored on the other side:

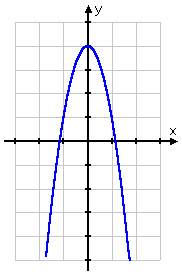
This mirroring virtually the y -centrality is a hallmark of fifty-fifty functions.

Also, I note that the exponents on all of the terms are fifty-fifty — the exponent on the constant term being zero: ivx ⁰ = four × 1 = 4. These are helpful clues that strongly propose to me that I've got an even function hither.

But the question asks me to make the determination algebraically, which means that I need to do the algebra.

And so I'll plug –x in for x , and simplify:

I can see, by comparing the original part with my final result in a higher place, that I've got a match, which ways that:

f(x) is fifty-fifty

---

• Determine algebraically whether f(x) = iix ³ – 4ten is even, odd, or neither.

If I graph this, I will see that it is "symmetric nigh the origin"; that is, if I start at a betoken on the graph on one side of the y -centrality, and draw a line from that signal through the origin and extending the same length on the other side of the y -axis, I will go to another point on the graph.

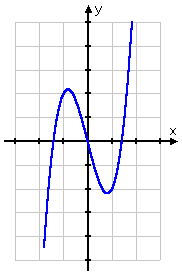
You tin also recollect of this every bit the one-half of the graph on 1 side of the y -axis is the upside-down version of the half of the graph on the other side of the y -axis. This symmetry is a hallmark of odd functions.

Note also that all the exponents in the part's rule are odd, since the second term can exist written as 4x = 4x ¹ . This is a useful inkling. I should expect this role to be odd.

The question asks me to make the decision algebraically, so I'll plug –x in for ten , and simplify:

f(–x) = 2(–ten)³ – four(–x)

= ii(–x ^three) + 4x

= –2x ³ + ivten

For the given role to exist odd, I need the in a higher place result to have all opposite signs from the original function. So I'll write the original function, then switch all the signs:

original: f(10) = 2(x)³ – 4(x)

switched: – f(x) = – 2x ^three + 4ten

Comparison this to what I got, I see that they're a lucifer. When I plugged –x in for ten , all the signs switched. This means that, as I'd expected:

f(ten) is odd.

---

• Determine algebraically whether f(x) = 210 ^three – 3x ^two – 4x + 4 is even, odd, or neither.

This function is the sum of the previous two functions. But, while the sum of an odd and an even number is an odd number, I cannot conclude the same of the sum of an odd and an even part.

Note that the graph of this role does non have the symmetry of either of the previous ones:

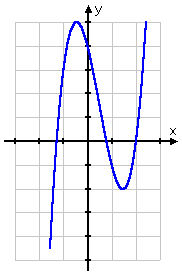
...nor are all of its exponents either fifty-fifty or odd.

Based on the exponents, as well as the graph, I would expect this function to be neither even nor odd. To be sure, though (and in gild to get full credit for my answer), I'll need to exercise the algebra.

I'll plug –x in for x , and simplify:

f(–x) = 2(–10)^three – 3(–ten)^two – 4(–ten) + iv

= 2(–x ^three) – three(x ²)  + 4x + 4

= –twoten ³ – 3x ² + ivx + 4

I can come across, by a quick comparing, that this does non lucifer what I'd started with, then this function is not fifty-fifty. What about odd?

To bank check, I'll write down the exact opposite of what I started with, existence the original function, only with all of the signs changed:

– f(x) = – 210 ³ + iii10 ² + fourx – 4

This doesn't match what I came upwardly with, either. And so the original function isn't odd, either. Then, as I'd expected:

f(x) is neither even nor odd.

---

As you lot can meet, the sum or deviation of an even and an odd function is not an odd function. In fact, y'all'll observe that the sum or divergence of two even functions is another even function, only the sum or divergence of two odd functions is another odd part.

At that place is (exactly) one function that is both even and odd; it is the zero function, f(x) = 0.

In other words, "even" and "odd", in the context of functions, hateful something every unlike from how these terms are used with whole numbers. Don't effort to mix the two sets of definitions; it'll only confuse you.

---

Just considering all of the examples so far have involved polynomial functions, don't think that the concept of even and odd functions is restricted to polynomials. It's not. Trigonometry is full of functions that are fifty-fifty or odd, and other types of functions can come under consideration, too.

• Determine whether is even, odd, or neither.

This is a rational function. The process for checking if information technology's even, odd, or neither is the aforementioned as e'er. I'll starting time past plugging –x in for x :

I can see, past comparing, that this is the same equally what I'd started with. Then:

g(x) is even

---

Yous may discover information technology helpful, when answering this "even or odd" type of question, to write down –f(x) explicitly, and then compare this to whatever you get for f(–ten). This can aid you make a confident determination of the correct answer.

Source: https://www.purplemath.com/modules/fcnnot3.htm

Posted by: sheltonhemperess.blogspot.com

Share this post